The author of this paper is Nick Szabo. He is polymath, computer sc...
### TL;DR
- Humans evolved in small, largely self-sufficient, an...
If you want to expand on this topic here are other interested resou...
how do i take a printout of this paper?
https://en.wikipedia.org/wiki/John_Maynard_Smith
> ***"money is a formal token of delayed reciprocal altruism" *** -...
### Yap, the island of stone money
Another interesting form of m...
Look up this source later.
### Fun fact:
**Barter doesn't scale.** Imagine that you could ...
Some examples of valued artifacts/collectibles:
 working for his brides.
Tribute may be the precursor to slavery since if you corral tribute...
### Laffer curve:
The Laffer curve was first introduced introduce...
**The first coins in human history were created around 640 BC by Ki...
For a given artifact to have value and be considered as a collectib...
Not discussed here is the importance of ornamentation for signaling...
### First human collectibles
Flints were likely the first human ...
#### Fiat currency:
Fiat money is currency that has no intrinsic v...
Shelling Out: The Origins of Money
Nick Szabo
Originally published in 2002
The precursors of money, along with language, enabled early modern humans to solve problems
of cooperation that other animals cannot - including problems of reciprocal altruism, kin altruism,
and the mitigation of aggression. These precursors shared with non-fiat currencies very specific
characteristics - they were not merely symbolic or decorative objects.
I. MONEY
From the very start, England’s 17th century colonies in
America had a problem - a shortage of coins [D94][T01]
The British idea was to grow large amounts of tobacco,
cut timber for the ships of their global navy and merchant
marine, and so forth, sending in return the supplies they
felt were needed to keep the Americans working. In ef-
fect, early colonists were supposed to both work for the
company and shop at the company store. The investors
and the Crown much preferred this to paying in coin what
the farmers might ask, letting the farmers themselves buy
the supplies - and, heaven forbid, keep some of the profit
as well.
The colonists’ solution was at hand, but it took a few
years for them to recognize it. The natives had money,
but it was very different from the money Europeans were
used to. American Indians had been using money for mil-
lenia, and quite useful money it turned out to be for the
newly arrived Europeans - despite the prejudice among
some that only metal with the faces of their political
leaders stamped on it constituted real money. Worse,
the New England natives used neither silver nor gold.
Instead, they used the most appropriate money to be
found in their environment - durable skeleton parts of
their prey. Specifically, they used wampum, shells of the
clam venus mercenaria and its relatives, strung onto pen-
dants.
FIG. 1: Necklace of wampum. During trade the beads were
counted, removed, and re-assembled on new necklaces. Native
American shell beads were also sometimes woven into belts or
other mnemonic and ceremonial devices that demonstrated
the wealth and commitment of a tribe to a treaty.
Clams were found only at the ocean, but wampum
traded far inland. Sea-shell money of a variety of
types could be found in tribes across the American con-
tinent. The Iriquois managed to collect the largest
wampum treasure of any tribe, without venturing any-
where near the clam’s habitat.[D94] Only a handful of
tribes, such as the Narragansetts, specialized in manufac-
turing wampum, while hundreds of other tribes, many of
them hunter-gatherers, used it. Wampum pendants came
in a variety of lengths, with the number of beads propor-
tional to the length. Pendants could be cut or joined to
form a pendant of length equal to the price paid.
Once they got over their hangup about what consti-
tutes real money, the colonists went wild trading for and
with wampum. Clams entered the American vernacular
as another way to say "money". The Dutch governor of
New Amsterdram (now New York) took out a large loan
from an English-American bank - in wampum. After a
while the British authorities were forced to go along. So
between 1637 and 1661, wampum became legal tender
in New England. Colonists now had a liquid medium of
exchange, and trade in the colonies flourished.[D94]
The beginning of the end of wampum came when the
British started shipping more coin to the Americas, and
Europeans started applying their mass-manufacturing
techniques. By 1661, British authorities had thrown in
the towel, and decided it would pay in coin of the realm -
which being real gold and silver, and its minting audited
and branded by the Crown, had even better monetary
qualities than shells. In that year wampum ceased to be
legal tender in New England. In 1710 briefly became le-
gal tender in North Carolina. It continued to be used as a
medium of exchange, in some cases into the 20th century
- but its value had been inflated one hundred fold by
Western harvesting and manufacturing techniques, and
it gradually went the route that gold and silver jewelry
had gone in the West after the invention of coinage -
from well crafted money to decoration. The American
language of shell money became a quaint holdover - "a
hundred clams" became "a hundred dollars". "Shelling
out" came to mean paying in coins or bills, and eventu-
ally by check or credit card.[D94] Little did we know that
we had touched the very origins of our species.
2
II. COLLECTIBLES
Native American money took many forms besides
shells. Furs, teeth, and a variety of other objects with
properties we will discuss below were also commonly used
as media of exchange. 12,000 years ago, in what is now
Washington state, the Clovis people developed some mar-
velously long chert blades. The only problem - they break
far too easily. They were useless for cutting. The flints
were being made "for the sheer enjoyment" - or for some
other purpose that had nothing to do with cutting.[G01]
As we shall see, this seeming frivolity was, quite likely,
actually very important to their survival.
Native Americans had not, however, been the first to
make artful but useless blades, nor had they invented
shell money. Nor, for that matter, had Europeans, even
though they, too, in ages past had widely used shells
and teeth for money - not to mention cattle, gold, silver,
weapons, and much else. Asians had used all that and
faux axes issued by governments to boot, but they as
well imported this institution. For archaeologists have
found pendants of shells dating to the early Paleolithic
that could easily have substituted for Native American
money.
FIG. 2: Beads made from shells of the pea-sized snail Nas-
sarius kraussianus, that lived in a nearby estuary. Blombos
Cave, South Africa, 75,000 B.P.[B04]
In the late 1990s archaeologist Stanley Ambrose dis-
covered, in the a rock-shelter in the Rift Valley of Kenya,
a cache of beads made of ostrich eggshell, blanks, and
shell fragments. They are dated using the argon-argon
(40Ar/39Ar) ratio to at least 40,000 years old[A98].
Pierced animal teeth have been found in Spain also dat-
ing to this time.[W95] Perforated shells have also been
recovered from early Paleolithic sites in Lebanon[G95].
Recently regular shells, prepared as strung beads and
dating further back still, to 75,000 BP, have been found
in Blombos Cave in South Africa.[B04]
Our modern subspecies had migrated to Europe and
necklaces of shell and tooth appear there, from 40,000
B.P. onward. Shell and tooth pendants appear in Aus-
tralia from 30,000 B.P. onward[M93]. In all cases, the
work is highly skilled, indicating a practice that probably
FIG. 3: Ostrich-eggshell beads, Kenya Rift Valley, 40,000 B.P.
(Courtesy Stanley Ambrose)
dates much further back in time. The origin of collecting
and decorating is quite likely Africa, the original home-
land of the anatomically modern subspecies. Collecting
and making necklaces must have had an important se-
lection benefit, since it was costly - manufacture of these
shells took a great deal of both skill and time during an
era when humans lived constantly on the brink of star-
vation[C94].
Practically all human cultures, even those that do not
engage in substantial trade or that use more modern
forms of money, make and enjoy jewelry, and value cer-
tain objects more for their artistic or heirloom qualities
than for their utility. We humans collect necklaces of
shells and other kinds of jewelry - for the sheer enjoy-
ment of it. For the evolutionary psychologists an expla-
nation that humans do something for "the sheer enjoy-
ment of it" is not an explanation at all - but the posing
of a problem. Why do so many people find the collection
and wearing of jewelry enjoyable? For the evolutionary
psychologist, this question becomes - what caused this
pleasure to evolve?
FIG. 4: Detail of necklace from a burial at Sungir, Russia,
28,000 BP. Interlocking and interchangeable beads. Each
mammoth ivory bead may have required one to two hours
of labor to manufacture.[W97]
III. EVOLUTION, COOPERATION, AND
COLLECTIBLES
Evolutionary psychology starts with a key mathemat-
ical discovery of John Maynard Smith[D89]. Using mod-
3
els of populations of co-evolving genes, from the well-
developed area of population genetics, Smith posited
genes that can code for strategies, good or bad, used
in simple strategic problems (the "games" of game the-
ory). Smith proved that these genes, competing to be
propagated into future generations, will evolve strategies
that are Nash equilbria to the strategic problems pre-
sented by the competition. These games include the pris-
oner’s dilemma, a prototypical problem of cooperation,
and hawk/dove, a prototypical problem of aggression and
its mitigation.
Critical to Smith’s theory is that these strategic games,
while played out between phenotypes proximately, are in
fact games between genes the ultimate level - the level
of competition to be propagated. The genes - not nec-
essarily the individuals - influence behavior as if they
were boundedly rational (coding for strategies as opti-
mal as possible, within the limits of what phenotypes
can express given the biological raw materials and pre-
vious evolutionary history) and "selfish" (to use Richard
Dawkins’ metaphor). Genetic influences on behavior are
adaptations to the social problems presented by genes
competing through their phenotypes. Smith called these
evolved Nash equilibria evolutionary stable strategies.
The "epicycles" built on top of the earlier individual
selection theory, such as sexual selection and kin selec-
tion, disappear into this more general model which, in a
Copernican manner, puts the genes rather than individu-
als at the center of the theory. Thus Dawkins’ metaphor-
ical and often misunderstood phrase, "selfish gene", to
describe Smith’s theory.
Few other species cooperate on the order of even Pale-
olithic humans. In some cases - brood care, the colonies
of ants, termites, and bees, and so forth, animals co-
operate because they are kin - because they can help
copies of their "selfish genes" found in their kin. In some
highly constrained cases, there is also ongoing coopera-
tion between non-kin, which evolutionary psychologists
call reciprocal altruism. As Dawkins describes it[D89],
unless an exchange of favors is simultaneous (and some-
times even then), one party or the other can cheat. And
they usually do. This is the typical result of a game
theorists call the Prisoner’s Dilemna - if both parties co-
operated, both would be better off, but if one cheats, he
gains at the expense of the sucker. In a population of
cheaters and suckers, the cheaters always win. However,
sometimes animals come to cooperate through repeated
interactions and a strategy called Tit-for-Tat: start coop-
erating and keep cooperating until the other party cheats
- then defect yourself. This threat of retalation motivates
continued cooperation.
The situations where such cooperation in fact occurs
in the animal world are highly constrained. The main
constraint is that such cooperation is restricted to rela-
tionships where at least one of the participants is more or
less forced to be in the proximity of the other. The most
common case is when parasites, and hosts whose bodies
they share, evolve into symbiotes. If the interests of the
parasite and the host coincide, so that both working to-
gether would be more fit than either on their own, (i.e.
the parasite is also providing some benefit to the host),
then, if they can play a successful game of Tit-for-Tat,
they will evolve into symbiosis - a state where their in-
terests, and especially the exit mechanism of genes from
one generation to the next, coincides. They become as a
single organism. However, there is much more than coop-
eration going on here - there is also exploitation. They
occur simultaneously. The situation is ananalogous to
an institution humans would develop - tribute - which
we will analyze below.
Some very special instances occur that do not involve
parasite and host sharing the same body and evolving
into symbiotes. Rather, they involve non-kin animals
and highly constrained territory. A prominent example
Dawkins describes are cleaner fish. These fish swim in
and out of the mouths of their hosts, eating the bacteria
there, benefiting the host fish. The host fish could cheat
- it could wait for the cleaner to finish its job, then eat
it. But they don’t. Since they are both mobile, they are
both potentially free to leave the relationship. However,
the cleaner fish have evolved a very strong sense of in-
dividual territoriality, and have stripes and dances that
are difficult to spoof - much like a difficult to forge brand
logo. So the host fish know where to go to get cleaned -
and they know that if they cheat, they will have to start
over again with a new distrustful cleaner fish. The en-
trance costs, and thus the exit costs, of the relationship
are high, so that it works out without cheating. Besides,
the cleaner fish are tiny, so the benefit of eating them is
not large compared to the benefit of a small number of,
or even one, cleaning.
One of the most pertinent examples.is the vampire bat.
As their name suggests, they suck the blood of prey mam-
mals. The interesting thing is that, on a good night, they
bring back a surplus; on a bad night, nothing. Their dark
business is highly unpredictable. As a result, the lucky
(or skilled) bats often share blood with the less lucky (or
skilled) bats in their cave. They vomit up the blood and
the grateful recipient eats it.
The vast majority of these recipients are kin. Out
of 110 such regurgitations witnessed by the strong-
stomached biologist G.S. Wilkinson, 77 were cases of
mothers feeding their children, and most of the other
cases also involved genetic kin. There were, however,
a small number that could not be explained by kin al-
truism. To demonstrate these were cases of reciprocal al-
4
truism, Wilkinson combined the populations of bats from
two different groups. Bats, with very rare exception, only
fed old friends from their original group.[D89] Such coop-
eration requires building a long-term relationship, where
partners interact often, recognize each other, and keep
track of each other’s behavior. The bat cave helps con-
strain the bats into long-term relationships where such
bonds can form.
We will see that some humans, too, chose highly risky
and discontinuous prey items, and shared the resulting
surpluses with non-kin. Indeed, they accomplished this
to a far greater extent than the vampire bat. How they
did so is the main subject of our essay. Dawkins suggests,
"money is a formal token of delayed reciprocal altruism",
but then pursues this fascinating idea no further. We will.
Among small human groups, public reputation can su-
percede retaliation by a single individual to motivate
cooperation in delayed reciprocation. However, reputa-
tional beliefs can suffer from two major kinds of errors
- errors of about which person did what, and errors in
appraising the value or damages caused by that act.
The need to remember faces and favors is a major cog-
nitive hurdle, but one that most humans find relatively
easy to overcome. Recognizing faces is easy, but remem-
bering that a favor took place when such memory needs
to be recalled can be harder. Remembering the specifics
about a favor that gave it a certain value to the favored
is harder still. Avoiding disputes and misunderstandings
can be improbable or prohibitively difficult.
The appraisal or value measurement problem is very
broad. For humans it comes into play in any system of
exchange - reciprocation of favors, barter, money, credit,
employment, or purchase in a market. It is important
in extortion, taxation, tribute, and the setting of judi-
cial penalties. It is even important in reciprocal altruism
in animals. Consider monkeys exchanging favors - say
pieces of fruit for back scratches. Mutual grooming can
remove ticks and fleas that an individual can’t see or
reach. But just how much grooming versus how many
pieces of fruit constitutes a reciprocation that both sides
will consider to be "fair", or in other words not a de-
fection? Is twenty minutes of backscratching worth one
piece of fruit or two? And how big a piece?
Even the simple case of trading blood for blood is more
complicated then it seems. Just how do the bats estimate
the value of blood they have received? Do they estimate
the value of a favor by weight, by bulk, by taste, by
its ability to satiate hunger, or other variables? Just
the same, measurement complications arise even in the
simple monkey exchange of "you scratch my back and I’ll
scratch yours".
For the vast majority of potential exchanges, the mea-
surement problem is intractible for animals. Even more
than the easier problem of remembering faces and match-
ing them to favors, the ability of both parties to agree
with sufficient accuracy on an estimate of the value of a
favor in the first place is probably the main barrier to
reciprocal altruism among animals.
Just the stone tool-kit of even early Paleolithic man
that has survived for us to find was in some ways too
complicated for brains of our size. Keeping track of favors
involving them - who manufactured what quality of tool
for whom, and therefore who owed whom what, and so
on - would have been too difficult outside the boundaries
of the clan. Add onto that, quite likely, a large variety of
organic objects, ephemeral services (such as grooming),
and so on that have not survived. After even a small
fraction of these goods had been transferred and services
performed our brains, as inflated as they are, could not
possibly keep track of who owed what to whom. Today
we often write these things down - but Paleolithic man
had no writing. If cooperation occured between clans
and even tribes, as the archaeological record indicates
in fact occured, the problem gets far worse still, since
hunter-gatherer tribes were usually highly antagonistic
and mutually distrustful.
If clams can be money, furs can be money, gold can be
money, and so on - if money is not just coins or notes is-
sued by a government under legal tender laws, but rather
can be wide variety of objects - then just what is money
anyway? And why did humans, often living on the brink
of starvation, spend so much time making and enjoying
those necklaces when they could have been dong more
hunting and gathering? Nineteenth century economist
Carl Menger[M1892] first described how money evolves
naturally and inevitably from a sufficient volume of com-
modity barter. In modern economic terms the story is
similar to Menger’s.
Barter requires a coincidence of interests. Alice grows
some pecans and wants some apples; Bob grows apples
and want some pecans. They just happen to have their
orchards near each other, and Alice just happens to trust
Bob enough to wait between pecan harvest time and ap-
ple harvest time. Assuming all these conditions are met,
barter works pretty well. But if Alice was growing or-
anges, even if Bob wanted oranges as well as pecans,
they’d be out of luck - oranges and apples don ’t both
grow well in the same climate. If Alice and Bob didn’t
trust each other, and couldn’t find a third party to be a
middleman[L94] or enforce a contract, they’d also be out
of luck.
Further complications could arise. Alice and Bob can’t
fully articulate a promise to sell pecans or apples in the
future, because, among other possibilities, Alice could
keep the best pecans to herself (and Bob the best ap-
ples), giving the other the dregs. Comparing the qual-
5
ities as well as the quantities of two different kinds of
goods is all the more difficult when the state of one of
the goods is only a memory. Furthermore, neither can
anticipate events such as a bad harvest. These compli-
cations greatly add to the problem of Alice and Bob de-
ciding whether separated reciprocal altruism has truly
been reciprocal. These kinds of complications increase
the greater the time interval and uncertainty between
the original transaction and the reciprocation.
A related problem is that, as engineers would say,
barter "doesn’t scale". Barter works well at small vol-
umes but becomes increasingly costly at large volumes,
until it becomes too costly to be worth the effort. If there
are n goods and services to be traded, a barter market
requires n
2
prices. Five products would require twenty-
five prices, which is not too bad, but 500 products would
require 250,000 prices, which is far beyond what is prac-
tical for one person to keep track of. With money, there
are only n prices - 500 products, 500 prices. Money for
this purpose can work either as a medium of exchange or
simply as a standard of value - as long as the number of
money prices themselves do not grow too large to memo-
rize or change too often. (The latter problem, along with
an implicit insurance "contract", along with the lack of
a competitive market may explain why prices were often
set by long-evolved custom rather than proximate nego-
tiation).
Barter requires, in other words, coincidences of supply
or skills, preferences, time, and low transaction costs. Its
cost increases far faster than the growth in the number of
goods traded. Barter certainly works much better than
no trade at all, and has been widely practiced. But it is
quite limited compared to trade with money.
Primitive money existed long before large scale trade
networks. Money had an even earlier and more impor-
tant use. Money greatly improved the workings of even
small barter networks by greatly reducing the need for
credit. Simultaneous coincidence of preference was far
rarer than coincidences across long spans of time. With
money Alice could gather for Bob during the ripening of
the blueberries this month, and Bob hunt for Alice during
the migration of the mammoth herds six months later,
without either having to keep track of who owed who, or
trust the other’s memory or honesty. A mother’s much
greater investment in child rearing could be secured by
gifts of unforgeable valuables. Money converts the divi-
sion of labor problem from a prisoner’s dilemma into a
simple swap.
The proto-money used by many hunter-gatherer tribes
looks very different from modern money, now serves a
different role in our modern culture, and had a function
probably limited to small trade networks and other lo-
cal institutions discussed below. I will thus call such
money collectibles instead of money proper. The terms
used in the anthropological literature for such objects
are usually either "money", defined more broadly than
just government printed notes and coins but more nar-
rowly than we will use "collectible" in this essay, or the
vague "valuable", which sometimes refers to items that
are not collectibles in the sense of this essay. Reasons for
choosing the term collectible over other possible names
for proto-money will become apparent. Collectibles had
very specific attributes. They were not merely sym-
bolic. While the concrete objects and attributes val-
ued as collectible could vary between cultures, they were
far from arbitrary. The primary and ultimate evolution-
ary function of collectibles was as a medium for storing
and transfering wealth. Some kinds of collectibles, such
as wampum, could be quite functional as money as we
moderns know it, where the economic and social condi-
tions encouraged trade. I will occasionally use the terms
"proto-money" and "primitive money" interchangeably
with "collectible" when discussing pre-coinage media of
wealth transfer.
IV. GAINS FROM WEALTH TRANSFERS
People, clans or tribes trade voluntarily because both
sides believe they gain something. Their beliefs about
the value may change after the trade, for example as they
gain experience with the good or service. Their beliefs at
the time of the trade, although to some degree inaccurate
as to the value, are still usually correct as to the existence
of gain. Especially in early intertribal trade, restricted
to high value items, there was strong incentive for each
party to get their beliefs right. Thus trade almost always
did benefit both parties. Trade created value as much as
the physical act of making something.
Because individuals, clans, and tribes all vary in their
preferences, vary in their ability to satisfy these pref-
erences, and vary in the beliefs they have about these
skills and preferences and the objects that are consequent
of them, there are always gains to be made from trade.
Whether the costs of making these trades - transaction
costs - are low enough to make the trades worthwhile
is another matter. In our civilization, far more trades
are possible than were through most of human history.
Nevertheless, as we shall see some kinds of trades were
worth more than the transaction costs, for some cultures,
probably back to the beginning of homo sapiens sapiens.
Voluntary spot trades are not the only kinds of trans-
actions that benefit from lower transaction costs. This
is the key to understanding the origin and evolution of
money. Family heirlooms could be used as collateral to
remove the credit risk from delayed exchanges. The abil-
6
ity of a victorious tribe to extract tribute from the van-
quished was of great benefit to the victor . The victor’s
ability to collect tribute benefited from some of the same
kinds of transaction cost techniques as did trade. So did
the plaintiff in assessment of damages for offenses against
custom or law, and kin groups arranging a marriage. Kin
also benefited from timely and peaceful gifts of wealth by
inheritance. The major human life events that modern
cultures segregate from the world of trade benefited no
less than trade, and sometimes more so, from techniques
that lowered transaction costs. None of these techniques
was more effective, important, or early than primitive
money - collectibles.
When H. sapiens sapiens displaced H. sapiens nean-
derthalis, population explosions followed. Evidence from
the takeover in Europe, c. 40,000 to 35,000 B.P, indicates
that H. sapiens sapiens increased the carrying capacity
of its environment by a factor of ten over H. sapiens ne-
anderthalis - i.e., the population density increased ten-
fold[C94]. Not only that, the newcomers had spare time
to create the world’s first art - such as the wonderful cave
paintings, a wide variety of well crafted figurines - and of
course the wonderful pendants and necklaces of seashells,
teeth, and eggshell.
These objects were not useless decorations. Newly ef-
fective wealth transfers, made possible by collectibles as
well as other probable advance of the era, language, cre-
ated new cultural institutions that quite likely played the
leading role in the increase of carrying capacity.
The newcomers, H. sapiens sapiens, had the same size
brain, weaker bones, and smaller muscles than the Ne-
anderthals. Their hunting tools were more sophisticated,
but in 35,000 B.P. they were basically the same tools
- they were probably not even twice as effective, much
less ten times more effective. The biggest difference may
have been wealth transfers made more effective or even
possible by collectibles. H. sapiens sapiens took pleasure
from collecting shells, making jewelry out of them, show-
ing them off, and trading them. H. sapiens neanderthalis
did not. The same dynamic would have been at work,
tens of thousands of years earlier, on the Serengeti, when
H. sapiens sapiens first appeared in that dynamic mael-
strom of human evolution, Africa.
We shall describe how collectibles lowered transaction
costs in each kind of wealth transfer - in the voluntary free
gift of inheritance, in voluntary mutual trade or marriage,
and in the involuntary transfers of legal judgments and
tribute.
All these kinds of value transfer occured in many cul-
tures of human prehistory, probably from the beginning
of Homo sapiens sapiens. The gains to be made, by one
or both parties, from these major life event transfers of
wealth, were so great that they occurred despite high
transaction costs. Compared to modern money, primi-
tive money had a very low velocity - it might be trans-
ferred only a handful of times in an average individual’s
lifetime. Nevertheless, a durable collectible, what today
we would call an heirloom, could persist for many gen-
erations and added substantial value at each transfer -
often making the transfer even possible at all. Tribes
therefore often spent large amounts of time on the seem-
ingly frivolous tasks of manufacturing and exploring for
the raw materials of jewelry and other collectibles.
V. THE KULA RING
FIG. 5: The Kula trading network of pre-colonial Melane-
sia. The kula valuables doubled as "high power" money
and mnemonic for stories and gossip. Many of the goods
traded, mostly agricultural products, were available in dif-
ferent seasons, and so could not be traded in kind. Kula
collectibles solved this double-coincidence problem as an un-
forgeabaly costly, wearable (for security), and circulated (lit-
erally!) money. Necklaces circulated clockwise, and armshells
counter-clockwise, in a very regular pattern. By solving the
double-coincidence problem an armshell or necklace would
prove more valuable than its cost after only a few trades, but
could circulate for decades. Gossip and stories that about
prior owners of the collectibles further provided information
about upstream credit and liquidity. In other Neolithic cul-
tures collectibles, usually shells, circulated in a less regular
pattern but had similar purposes and attributes.[L94]
7
FIG. 6: Kula armshell (mwali).
FIG. 7: Kula necklaces (bagi).
For any institution in which wealth transfer is an im-
portant component, we will ask the following questions:
1 - What coincidence in time between the event, the
supply for the transfered good, and demand for the trans-
fered good was necessary? How unlikely or how high a
barrier to the wealth transfer did the improbability of
coincidence represent?
2 - Would the wealth transfers formed a closed loop of
collectibles just based on that institution, or were other
wealth transfer institutions necessary to complete circu-
lation cycles? Taking the actual flow graph of mone-
tary circulation seriously is critical to understanding the
emergence of money. General circulation among a wide
variety of trades did not and would not exist for most
of human prehistory. Without completed and repeated
loops collectibles would not circulate and would become
worthless. A collectible, to be worth making, had to add
value in enough transactions to amortize its cost.
We shall first examine the kind of transfer most famil-
iar and economically important to us today - trade.
VI. STARVATION INSURANCE
Bruce Winterhalder[W98] surveys models of how and
why food is sometimes transfered between animals: tol-
erated theft, producing/scrounging/opportunism, risk-
sensitive subsistence, by-product mutualism, delayed
reciprocity, trade/exchange not in kind, and other selec-
tion models (including kin altruism). Here we focus on
risk-sensitive subsistence, delayed reciprocity, and trade
(exchange not in kind). We argue that substituting trade
of food for collectibles for delayed reciprocity can increase
food sharing. It does so by mitigating the risks of a vari-
able food supply while avoiding the largely insurmount-
able problems of delayed reciprocity between bands. We
will deal with kin altruism and theft (tolerated or not)
in broader contexts below.
Food is worth far more to starving people than to well
fed ones. If the starving man can save his life by trad-
ing his most precious valuables, it may be worth to him
months or even years of the labor it might take to re-
place that value. He will usually consider his life worth
more than the sentimental value of the family heirlooms.
Like fat itself, collectibles can provide insurance against
food shortages. Starvation from local shortages could be
staved off with at least two different kinds of trades - for
the food itself, or for foraging or hunting rights.
Nevertheless, the transaction costs were usually too
high - bands were far more likely to fight than ever trust
each other. The hungry band that couldn’t find its own
food usually starved. However, if the transaction costs
could be lowered, by lowering the need for trust between
bands, food that was worth a day’s labor to one band
might be worth several months’ labor to the starving
band.
Local but extremely valuable trade was, this essay ar-
gues, made possible among many cultures by the ad-
vent of collectibles, by the time of the Upper Paleolithic.
Collectibles substituted for otherwise necessary but non-
existent trusting long term relationships. If there had
existed a high degree of sustained interaction and trust
between tribes, or individuals of different tribes, so that
they gave each other unsecured credit, this would have
stimulated time-lagged barter trade. However, such a
high degree of trust then is highly implausible - for
the reasons stated above regarding reciprocal altruism,
confirmed by the empirical evidence that most hunter-
gatherer tribal relations have been observed to be quite
antagonistic. Hunter-gatherer bands usually broke up
into small bands for most of the year and gathered into
"aggregates", something like medieval European fairs, for
a few weeks out of the year. Despite the lack of trust be-
tween bands, an important trade in staples, of the kind
illustrated in the accompanying figure, almost surely oc-
8
curred in European and probably elsewhere, such as with
the big game hunters of America and Africa.
The scenario illustrated by the accompanying figure
is hypothetical, but it would be very surprising if it did
not occur. While many Europeans even in the Paleolithic
enjoyed wearing shell necklaces, many lived farther inland
and made necklaces instead out of the teeth of their prey.
Flints, axes, furs, and other collectibles were also quite
likely used as media of exchange.
Reindeer, bison, and other human prey migrated at dif-
ferent times of the year. Different tribes specialized in dif-
ferent prey, to the point where over 90%, and sometimes
as much as 99%, of the remains from many sites during
the Paleolithic in Europe come from a single species[C94].
This indicates at least seasonal specialization and per-
haps full-time specialization by a tribe in a single species.
To the extent they specialized, the members of a single
tribe would have become experts at the behavior, migra-
tion habits, and other patterns surrounding their specific
prey species, as well as the specialized tools and tech-
niques for hunting them. Some tribes observed in re-
cent times are known to have specialized. Some North
American Indian tribes specialized respectively in hunt-
ing bison, antelope, and fishing for salmon. In northern
Russia and parts of Finland, many tribes, including the
Lapp even today, specialized in herding a single species
of reindeer.
Such specialization was probably far higher when more
large prey (horse, auroch, giant elk, bison, giant sloth,
mastadon, mammoth, zebra, elephant, hippopotamus,
giraffe, musk oxen, etc.) roamed North America, Eu-
rope, and Africa in large herds during the Paleolithic.
Large wild animals unafraid of humans no longer exist.
During the Paleolithic they were either driven extinct
or adapted to be afraid of humans and our projectiles.
However, for most of the time span of H. sapiens sapi-
ens these herds were abundant and easy pickings to spe-
cialist hunters. According to our theory of trade-based
predation, specialization was quite likely far higher when
large prey roamed North America, Europe, and Africa
in large herds during the Paleolithic. Trade-based divi-
sion of labor in hunting between tribes is consistent with
(although not securely confirmed by) the archaeological
evidence from the Paleolithic in Europe.
These migrating bands, following their herds, fre-
quently interacted, creating many opportunities for
trade. American Indians preserved food by drying, mak-
ing pemmican, and so on in ways that lasted for a few
months but typically not a full year. Such food was
commonly traded, along with skins, weapons, and col-
lectibles. Often these trades occurred during annual trad-
ing expeditions[T01].
Large herd animals migrated through a territory only
two times a year, with a window most often of one or
two months. Without any other source of protein besides
their own prey species, these specialist tribes would have
starved. The very high degree of specialization demon-
strated in the archaeological record could only have oc-
curred if there was trade.
Thus, even if the time-offset barter of meat were the
only kind of trade, this is quite sufficient to make the
use of collectibles quite worthwhile. The necklaces, flints,
and any other objects used as money circulate in a closed
loop, back and forth, in roughly equal amounts so long
as the value of meat traded remains roughly equal. Note
that it is not enough, for the theory of collectibles put
forth in this paper to be correct, that single beneficial
trades were possible. We must identify closed loops of
mutually beneficial trades. With closed loops the col-
lectibles continue to circulate, amortizing their costs.
As mentioned, we know from archaeological remains
that many tribes specialized in a single large prey species.
This specialization was at least seasonal; if there was
extensive trade it could have been full-time. Becoming
experts in the habits and migration patterns, and best
methods of taking down, a tribe reaped enormous pro-
ductive benefits. These benefits, however, would nor-
mally be unattainable, for specializing in a single species
meant going without food most of the year. Division of
labor between tribes paid off - and trade made it possible.
The supply of food would nearly double from trade just
between two complementary tribes. There were, how-
ever, rather than two prey species, often up to a dozen
that migrated through most hunting territories in areas
like the Serengeti and the European steppe. The amount
of meat available to a species-specializing tribe would
thus likely more than double with such trade among a
handful of neighboring tribes. On top of this, the extra
meat would be there when needed most - when the meat
from a tribe’s own species prey would already have been
eaten, and without food the hunters would starve.
Thus there were at least four gains, or sources of sur-
plus, from a trade cycle as simple as two prey species and
two non-simultaneous but offsetting trades. These gains
are distinct but not necessarily independent:
1 - An available source of meat at a time of the year
when one would otherwise starve.
2 - An increase in the total supply of meat - they traded
the surplus beyond what they could eat immediately or
store; what they didn’t trade would have gone to waste.
3 - An increase in the variety of nutrition from meat,
by eating different kinds of meat.
4 - Increased productivity from specialization in a sin-
gle prey species.
Making or saving collectibles to trade for food itself was
not the only way to insure against bad times. Perhaps
9
even more common, especially where large prey items
were not available, was territoriality combined with trade
in foraging rights. This can be observed even in some of
the remnants of hunter-gatherer culture that exist today.
The !Kung San of southern Africa, like all other
modern remnants of hunter-gatherer cultures, live on
marginal lands. They have no opportunity to be spe-
cialists but must take the meager remnants available.
They may thus be rather uncharacteristic of many an-
cient hunter-gatherer cultures, and uncharacteristic of
the original Homo sapien sapiens, which first seized the
lushest lands and best game routes from Homo sapi-
ens neanderthalis and only much later drove the Nean-
derthals from marginal lands. Yet despite their severe
ecological handicap, the !Kung use collectibles as items
of trade.
Like most hunter-gatherers, the !Kung spend most of
the year in small, dispersed bands and a few weeks of the
year in an aggregate with several other bands. Aggrega-
tion is like a fair with added features - trade is accom-
plished, alliances are cemented, partnerships strength-
ened, and marriages transacted. Preparation for aggre-
gation is filled with the manufacture of tradeable items,
partly utilitarian but mostly of a collectible nature. The
exchange system, called by the !Kung hxaro, involves a
substantial trade in beaded jewelry, including ostrich-
shell pendants quite similar to those found in Africa
40,000 years ago.
FIG. 8: Pattern of hxaro exchanges and kinship relations
among neighboring tribes of !Khung San hunter-gatherers.
One of the main things the !Kung buy and sell with
their collectibles are abstract rights to enter another
band’s territory and hunt or gather food there. Trade
FIG. 9: Necklaces used in the hxaro exchange.
in these rights is especially brisk during local shortages
which can be alleviated by foraging in a neighbor’s terri-
tory[W77][W82] !Kung bands mark their territories with
arrows; trespassing without having purchased the right
to enter and forage is tantamount to a declaration of war.
Like the inter-band food trade discussed above, the use
of collectibles to purchase foraging rights constitutes an
"insurance policy against starvation", to use the phrase
of Stanley Ambrose[A98].
Although anatomically modern humans surely had
conscious thought, language, and some ability to plan, it
would have required little conscious thought or language,
and very little planning, to generate trades. It was not
necessary that tribe members reasoned out the benefits
of anything but a single trade. To create this institution
it would have sufficed that people follow their instincts to
make obtain collectibles with the characteristics outlined
below. (as indicated by proxy observations that make
approximate estimations for these characteristics). This
is to various extents true of the other institutions we will
study - they evolved, rather than being consciously de-
signed. No one participating in the institution’s rituals
would have explained their function in terms of ultimate
10
evolutionary function; rather they explained in terms of
a wide variety of mythologies that served more as proxi-
mate motivators of behavior than as theories of ultimate
purpose or origin.
Direct evidence for trade in food has long since de-
cayed. We may, in the future, find more direct evidence
than is now available for this article, via comparison of
hunting remains in one tribe with the consumption pat-
terns in another tribe - the hardest part of this task likely
being to identify the boundaries of different tribes or kin
groups. According to our theory, such transfer of meat
from one tribe to another was common in many parts
of the world during the Paleolithic where large-scale and
specialized big game hunting occurred.
For now, we do have extensive indirect evidence of
trade, via the movement of the collectibles themselves.
Fortunately there is a good correlation between the dura-
bility desired for collectibles and the conditions under
which an artifact has survived to be found by today’s
archaeologists. In the early Paleolithic, when all human
movement was on foot, we have instances of perforated
sea shells found up to 500 kilometers away from the near-
est source[C94]. There was a similar long-distance move-
ment of flint.
Unfortunately, trade was severely restricted by high
transaction costs in most times and places. The pri-
mary barrier was the antagonism between tribes. The
predominate relationship between tribes was one of dis-
trust on good days and outright violence on bad days.
Only ties of marriage or kinship could bring tribes into
a relationship with trust, albeit only occasionally and of
limited scope. The poor ability to protect property, even
collectibles worn on the person or buried in well-hidden
caches, meant that collectibles had to amortize their costs
in a few transactions.
Trade was thus not the only kind of wealth trans-
fer, and probably not the most important kind dur-
ing the long human prehistory where high transaction
costs prevented the development of the kinds of markets,
firms, and other economic institutions we now take for
granted[L94]. Underneath our great economic institu-
tions are far more ancient institutions that also involved
wealth transfer - in prehistoric times, the main kinds of
wealth transfer. All of these institutions distinguished
Homo sapiens sapiens from previous animals. We now
turn to one of the most basic kinds of wealth transfer
that we humans take for granted but other animals do
not have - passing wealth onto the next generation.
VII. KIN ALTRUISM BEYOND THE GRAVE
Coincidence in time and locale of supply and demand
for trade was rare - so much so, that most kinds of trades
and trade-based economic institutions we now take for
granted could not exist. Even more unlikely was the
triple coincidence of supply with demand with a major
event for a kin group - the formation of a new family,
death, crime, or victory or defeat in war. As we shall
see, clans, and individuals greatly benefited from a timely
transfer of wealth during these events. Such wealth trans-
fer in turn was much less wasteful when it was the trans-
fer of a store of wealth more durable and general than
consumables or tools designed for other purposes. The
demand for a durable and general store of wealth for use
in these institutions was thus even more urgent than for
trade itself. Furthermore, the institutions of marriage,
inheritance, dispute resolution, and tribute may predate
intertribal trade, and involved for most tribes a greater
transfer of wealth than trade. These institutions thus
more than trade served as the motivator and incubator
of the earliest primitive money.
In most hunter-gatherer tribes this wealth came in a
form that strikes us preposterously wealthy moderns as
trivial - a collection of wooden utensils, flint and bone
tools and weapons, shells on strings, perhaps a hut and in
colder climates some mangy furs. Sometimes it could all
be carried on the person. Nevertheless, these motley as-
sortments were wealth for a hunter-gatherer no less than
real estate, stocks, and bonds are wealth for us. To the
hunter-gatherer tools and sometimes warm clothes were
necessary for survival. Many of the items were highly
valued collectibles that insured against starvation, pur-
chased mates, and could substitute for massacre or star-
vation in event of war and defeat. The ability to transfer
the capital of survival to one’s descendants was another
advantage Homo sapiens sapiens had over previous ani-
mals. Furthermore, the skilled tribesman or clan could
accumulate a surplus of wealth from the occasional, but
cumulative over a lifetime, trade of surplus consumables
for durable wealth, especially collectibles. A temporary
fitness advantage could be translated into a more durable
fitness advantage for one’s descendants.
Another form of wealth, hidden from the archaeolo-
gist, were titles to offices. Such social positions were
more valuable than the tangible forms of wealth in many
hunter-gatherer cultures. Examples of such positions in-
cluded clan leaders, war party leaders, hunting party
leaders, membership in a particular long-term trading
partnership (with a particular person in a neighboring
clan or tribe), midwives, and religious healers. Often
collectibles not only embodied wealth, but also served
as a mnemonic, representing the title to a clan position
11
of responsibility and privilege. Upon death, to maintain
order, the heirs to such positions had to be quickly and
clearly determined. Delays could spawn vicious conflicts.
Thus, a common event was the mortuary feast, in which
the deceased was feted while both his tangible and intan-
gible forms of wealth were distributed to descendants, as
determined by custom, clan decision-makers, or the will
of the deceased.
Other kinds of free gifts were quite rare in pre-modern
cultures, as Marcel Mauss[M50] and other anthropolo-
gists have pointed out. Seemingly free gifts in fact im-
plicitly invoked an obligation in the recipient. Before
contract law, this implicit obligation of the "gift", along
with community dishonor and punishments ensuing if the
implicit obligation was not met, was perhaps the most
common motivator of reciprocation in delayed exchange,
and is still common in the variety of informal favors we
do for each other. Inheritance and other forms of kin
altruism were the only widely practiced forms of what
we moderns would call gift proper, namely a gift that
imposed no obligation on the recipient.
Early Western traders and missionaries, who often saw
natives as childish primitives, sometimes called their trib-
ute payments "gifts" and trades "gift exchanges", as if
they bore more resemblance to the Christmas and birth-
day present exchanges of Western children than to the
contractual and tax obligations of adults. Partly this
may have reflected prejudice, and partly the fact that in
the West by that time obligations were usually formal-
ized in writing which the natives lacked. Westerners thus
usually translated the rich variety of words natives had
for their exchange institutions, rights, and obligations as
"gift". Seventeenth century French settlers in America
were thinly scattered among much larger populations of
Indian tribes, and often found themselves paying tribute
to these tribes. Calling these payments "gifts" was a way
for them to save face with other Europeans who faced no
such necessity and found it cowardly.
Mauss and modern anthropologists have unfortunately
kept this terminology. The uncivilized human is still like
a child, but now innocent like a child, a creature of moral
superiority who would not stoop to our kind of base, cold-
blooded economic transactions. However in the West,
especially in the official terminology used for our laws
covering transactions, a "gift" refers to a transfer that
imposes no obligation. When coming across anthropo-
logical discussions of "gift exchange" these caveats should
be kept in mind - modern anthropologists are not at all
referring to the free or informal gifts we commonly re-
fer to in our modern use on the term "gift". They are
rather referring to any of a wide variety of often quite
sophisticated systems of rights and obligations involved
in wealth transfers. The only major transactions in pre-
historic cultures similar to our modern gift, in that it was
neither itself a widely recognized obligation nor imposed
any obligation on the recipient, were parents or maternal
kin caring for their children and inheritance. (An excep-
tion was that inheriting title to a position imposed the
responsibilities of the position on the heir as well as its
privileges).
Inheritance of some heirlooms might proceed for sev-
eral generations uninterrupted, but it did not by itself
form a closed loop of collectibles transfers. Heirlooms
were only valuable if they eventually got used for some-
thing else. They often were used in marriage transactions
between clans that could form closed loop cycles of col-
lectibles.
VIII. THE FAMILY TRADE
An early and important example of a small closed loop
trade network made possible by collectibles involves the
much higher investment humans make in raising offspring
than our primate relatives, and the related human insti-
tution of marriage. Combining arrangements of long-
term matches for mating and child-raising, negotiated
between clans, with wealth transfer, marriage is a hu-
man universal and probably dates back to the first Homo
sapiens sapiens.
Parental investment is a long-term and almost one-
shot affair - there is no time for repeated interactions.
Divorce from a negligent father or unfaithful wife usually
represented several years of time wasted, in genetic fitness
terms, by the jilted party. Fidelity and commitment to
the children were primarily enforced by in-laws - the clan.
The marriage was the contract between clans that usually
included such promises of fidelity and commitment as
well as wealth transfer.
The contributions a man and a woman will bring to
a marriage are seldom equal. This was even more true
in an era when mate choice was largely determined by
clans and the population from which clan leaders could
choose was quite small. Most commonly, the woman was
considered more valuable and the groom’s clan paid a
bride price to the brides clan. Quite rare in compari-
sion was dowry, a payment by the bride’s clan to the
new couple. Mostly this was practiced by upper classes
of monogamous but highly unequal socieities in medieval
Europe and India, and was ultimately motivated by the
far greater reproductive potential of upper class sons than
upper class daughters in those societies. Since litera-
ture was mostly written about upper classes, dowry of-
ten plays a role in European traditional stories. This does
not reflect its actual frequency across human cultures -
it was quite rare.
12
Marriages between clans could form a closed cycle of
collectibles. Indeed, two clans exchanging partners would
be sufficient to maintain a closed loop, as long as brides
tended to alternate. If one clan was wealthier in col-
lectibles from some other kind of transfer, it could marry
more of its sons to better brides (in monogamous soci-
eties) or a greater number of brides (in polygamous so-
cieties). In a loop involving only marriages, primitive
money would simply serve to replace the need for mem-
ory and trust between clans over a long period of delay
between unbalanced transfers of reproductive resources.
Like inheritance, lawsuit, and tribute, marriage re-
quires a triple coincidence of the event, in this case the
marriage, with supply and demand. Without a transfer-
able and durable store of value, the current ability of a
groom’s clan to supply the current desires of the bride’s
clan, to a large enough degree to make up the value mis-
match between bride and groom, while also satisfying
the political and romantic constraints of the match, were
quite unlikely to be well satisfied. One solution is im-
posing an ongoing service obligation from the groom or
his clan to the bride’s clan. This occurs in about 15% of
known cultures[DW88]. In a much larger number, 67%,
the groom or groom’s clan pays the brides clan a substan-
tial amount of wealth. Some of this bride price is paid
in immediate consumables, in plants to be gathered har-
vested and animals slaughtered for the marriage feast. In
herding or agricultural societies much of the bride price is
paid in livestock, a long lasting form of wealth. The bal-
ance, and usually the most valuable portion of the bride-
price in cultures without livestock, is paid with what are
usually the most valuable family heirlooms - the rarest,
costliest, and most durable pendants, rings, and so on.
The Western practice of the groom giving the bride a ring
- and a suitor giving a maiden other kinds of jewelry - was
once a substantial transfer of wealth and was common in
many other cultures. In about 23% of cultures, mostly
modern ones, there is no substantial wealth exchange. In
about 6% of cultures there is mutual exchange of sub-
stantial wealth between bride and groom clans. In only
about 2% of cultures does the bride’s clan pay the new
couple a dowry.[DW88]
Unfortunately, some wealth transfers were a far cry
from the altruism of the inheritance gift or the joy of
marriage. Quite the opposite, in the case of tribute.
IX. THE SPOILS OF WAR
Death rates from violence in chimp troops and hunter-
gatherer human cultures alike are far higher than in mod-
ern civilizations. This probably dates at least as far back
as our common ancestor with the chimpanzees - chimp
troops, as well, are constantly fighting.
Warfare involved, among other things, killing, maim-
ing, torture, kidnapping, rape, and the extortion of trib-
ute in exchange for avoiding such fates. When two neigh-
bor tribes were not at war, one was usually paying tribute
to the other. Tribute could also serve to bind alliances,
achieving economies of scale in warfare. Mostly, it was
a form of exploitation more lucrative to the victor than
further violence against the defeated.
Victory in war was sometimes followed by an immedi-
ate payment from the losers to the victims. Often this
just took the form of looting by the enthusiastic vic-
tors, while the losers desperately hid their collectibles.
More often, tribute was demanded on a regular basis. In
this case, the triple coincidence could and sometimes was
avoided by a sophisticated schedule of payments in kind
that matched the losing tribe’s ability to supply a good
or service with the victor’s demand for it. However, even
with this solution primitive money could provide a better
way - a common medium of value that greatly simplified
the terms of payment - very important in an era when
terms of the treaty could not be recorded but had to be
memorized. In some cases, as with the wampum as used
in the Iriquois Confederacy, the collectibles doubled as
a primitive mnemonic device that, while not verbatim,
could be used as an aid to recall the terms of the treaty.
For the winners, collectibles provided a way to collect
tribute at closer to the Laffer optimum. For the losers,
collectibles buried in caches provided a way to "under-
report", leading the victors to believe the losers were less
wealthy and thus demand less than they might. Caches of
collectibles also provided insurance against over-zealous
tribute collectors. Much of the wealth in primitive soci-
eties escaped the notice of the missionaries and anthro-
pologists due to its highly secretive nature. Only arche-
ology can reveal the existence of this hidden wealth.
Hiding and other strategies presented a problem that
tribute collectors share with modern tax collectors - how
to estimate the amount of wealth they can extract. Value
measurement is a thorny problem in many kinds of trans-
actions, but never more so than in the antagonistic col-
lection of tax or tribute. In making these very difficult
and nonintuitive trade-offs, and then executing them in
a series of queries, audits, and collection actions, tribute
collectors efficiently optimized their revenue, even if the
results seemed quite wasteful to the tribute payer.
Imagine a tribe collecting tribute from several neigh-
bor tribes it previously defeated in war. It must estimate
how much it can extract from each tribe. Bad estimates
leave the wealth of some tribes understated, while forc-
ing others to pay tribute based on estimates of wealth
they don’t actually have. The result: the tribes that are
hurt tend to shrink. The tribes that benefit pay less trib-
13
ute than could be extracted. In both cases, less revenue
is generated for the victors than they might be able to
get with better rules. This is an application of the Laffer
curve to the fortunes of specific tribes. On this curve, ap-
plied to income taxes by the brilliant economist Arthur
Laffer, as the tax rate increases, the amount of revenue
increases, but at an increasingly slower rate than the tax
rate, due to increased avoidance, evasion, and most of
all disincentive to engage in the taxed activity. At a cer-
tain rate due to these reasons tax revenues are optimized.
Hiking the tax rate beyond the Laffer optimum results in
lower rather than higher revenues for the government.
Ironically, the Laffer curve was used by advocates for
lower taxes, even though it is a theory of tax collection
optimum to government revenue, not a theory of tax col-
lection optimal to social welfare or individual preference
satisfaction.
On a larger scale, the Laffer curve may be the most
important economic law of political history. Charles
Adams[A90] uses it to explain the rise and fall of empires.
The most successful governments have been implicitly
guided by their own incentives - both their short-term
desire for revenue and their long-term success against
other governments - to optimize their revenues accord-
ing to the Laffer Curve. Governments that overburdened
their taxpayers, such as the Soviet Union and later Ro-
man Empire, ended up on the dust-heap of history, while
governments that collected below the optimum were of-
ten conquered by their better-funded neighbors. Demo-
cratic governments may maintain high tax revenues over
historical time by more peaceful means than conquer-
ing underfunded states. They are the first states in his-
tory with tax revenues so high relative to external threats
that they have the luxury of spending most of the money
in non-military areas. Their tax regimes have operated
closer to the Laffer optimum than those of most previous
kinds of governments. (Alternatively, this luxury may
be made possible by the efficiency of nuclear weapons
in detering attack rather than the increased incentives
of democracies to optimize to tax collection). When we
apply the Laffer curve to examining the relative impact
of treaty tributterms on various tribes, we conclude that
the desire to optimize revenues causes victors to want to
accurately measure the income and wealth of the van-
quished. Measuring value is crucial to determining the
tributaries’ incentives to avoid or evade the tribute by
hiding wealth, fight, or flight. For their part, tributaries
can and do spoof these measurements in various ways,
for example by burying collectibles in caches. Tribute
collection involves a measurement game with unaligned
incentives.
With collectibles, one can demand tribute at strategi-
cally optimal times instead of when items can be supplied
by the tributary or is in demand by the victor. The vic-
tors can then choose when they will in the future consume
the wealth, rather than having to consume it at the time
the tribute is extracted. Much later, well into the dawn of
history, in 700 BC, though trade was widespread, money
still took the form of collectibles - made out of more pre-
cious metals, but in their basic characteristics, such as
lack of uniform value, similar to most of the proto-money
used since the dawn of Homo sapiens sapiens. This was
changed by a Greek-speaking culture in Anatolia (mod-
ern Turkey), the Lydians. Specifically, the kings of Lydia
were the first major issuers of coins in the archaeological
and historical record.
From that day to this, government mints with self-
granted monopolies, rather than private mints, have been
the main issuers of coin. Why wasn’t minting domi-
nated by private interests, such as private bankers, which
did exist at the time in these semi-market economies?
The main explanation for government dominance of coin
minting has been that only governments could enforce
anti-counterfeiting measures. However, they could have
enforced such measures in protection of competing pri-
vate mints, just as they enforce trademarks today and at
that time as well.
It was far easier to estimate the value of a coin than
that of a collectible - especially at low transaction values.
Far more trades could be made with money instead of
barter; indeed many kinds of low-value trades became
possible for the first time as the small gains from trade
for the first time exceeded transaction costs. Collectibles
were low velocity money, involved in a small number of
high value transactions. Coins were high velocity money,
facilitating a large number of low value trades.
Given what we have seen about the benefits of proto-
money to tribute and tax collectors, as well as the critical
nature of the value measurement problem in optimally
coercing such payments, it is not surprising that tax col-
lectors, specifically the kings of Lydia, were the first ma-
jor issuers of coinage. The king, deriving his revenue from
tax collection, had a strong incentive to measure to value
of wealth held and exchanged by his subjects more ac-
curately. That the exchange also benefited from cheaper
measurement by traders of the medium of exchange, cre-
ating something closer to efficient markets, and allowing
individuals to enter into the marketplace on a larger scale
for the first time, was for the king a fortuitous side ef-
fect. The greater wealth flowing through markets, now
available to be taxed, boosted the king’s revenues even
beyond the normal Laffer curve effect of reducing mis-
measurement between given tax sources.
This combination of more efficient tax collection with
more efficient markets meant a vast increase in overall tax
revenues. These tax collectors almost literally hit a gold
14
mine, and the wealth of Lydian kings Midas, Croesus,
and Giges is famous to this day.
A few centuries later, the Greek king Alexander the
Great conquered Egypt, Persia and much of India, fund-
ing his spectacular conquest by plundering Egyptian and
Persian temples, filled with assemblages of low-velocity
collectibles, and melting them down into high-velocity
coins. More efficient and encompassing market economies
as well as more efficient tax collection sprung up in his
wake.
Tribute payments did not form by themselves a closed
loop of collectibles. These were only valuable if they
ultimately could be used by the victors for something
else, such as marriage, trade, or collateral. However, vic-
tors could coerce the vanquished into manufacturing for
obtaining collectibles, even if it did not serve the van-
quished’s voluntary interests.
X. DISPUTES AND REMEDIES
Ancient hunter-gatherers did not have our modern tort
or criminal law, but they did have an analogous means
of settling disputes, often judged by clan or tribal lead-
ers or vote, that covered what modern law calls crimes
and torts. Settling disputes through punishments or pay-
ments sanction by the clans of the disputing parties sub-
stituted for cycles of revenge or vendetta wars. Most
pre-modern cultures, ranging from the Iriquois in Amer-
ica to the pre-Christian Germanic peoples, decided that
payment was better than punishment. Prices (e.g. the
Germanic "weregeld" and Iriquois blood money) were as-
signed to all actionable offenses, ranging from petty theft
to rape to murder. Where money was available, the pay-
ment took the form of money. Livestock was used in
herding cultures. Otherwise, payment of collectibles were
the most commonly used remedy.
The payment of remedies for damages in a lawsuit or
similar complaint led to the same kind of problem of
triple coincidence of event, supply, and demand as oc-
curred in inheritance, marriage, and tribute. The judg-
ment of the case had to coincide with the ability of the
plaintiff to pay the damages as well as the opportunity
and desire of the defendant to benefit from them. If the
remedy was a consumable the plaintiff already had plenty
of, the remedy still served as a punishment but would not
likely satisfy the defendant - and thus would not curb the
cycle of violence. Thus, we here again the value added by
collectibles - in this case, in making possible the remedy
to resolve a dispute or terminate a cycle of revenge.
Dispute remedies would not form a closed loop if the
payments served to entirely eliminate vendettas. How-
ever, if the payments did not completely damp the
vendetta, the payments could form a cycle following the
cycle of revenge. For this reason, it is possible that the
institution reached an equilibrium when it had reduced
but not eliminated cycles of revenge until the advent of
more densely connected trading networks.
XI. ATTRIBUTES OF COLLECTIBLES
Since humans evolved in small, largely self-sufficient,
and mutually antagonistic tribes, the use of collectibles to
reduce the need for favor-tracking, and to make possible
the other human institutions of wealth transfer we have
explored, was far more important than the scale problems
of barter for most of the timespan of our species. Indeed,
collectibles provided a fundamental improvement to the
workings of reciprocal altruism, allowing humans to co-
operate in ways unavailable to other species. For them,
reciprocal altruism is severely limited by unreliable mem-
ory. Some other species have large brains, build their own
homes, or make and use tools. No other species has pro-
duced such an improvement to the workings of reciprocal
altruism. The evidence indicates this new development
had matured by 40,000 B.P.
Menger called this first money an "intermediate com-
modity" - what this paper calls collectibles. An artifact
useful for other things, such as cutting, could also be
used as a collectible. However, once institutions involv-
ing wealth transfer became valuable, collectibles would be
manufactured just for their collectible properties. What
are these properties? For a particular commodity to be
chosen as a valuable collectible, it would have had, rela-
tive to products less valuable as collectibles, at least the
following desirable qualities:
1 - More secure from accidential loss and theft. For
most of history this meant carriable on the person and
easy to hide.
2 - Harder to forge its value. An important subset of
these are products that are unforgeably costly, and there-
fore considered valuable, for reasons explained below.
3 - This value was more accurately approximated by
simple observations or measurements. These observa-
tions would have had more reliable integrity yet have
been less expensive.
Humans the world over are strongly motivated to col-
lect items that better satisfy these properties. Some of
this motivation probably includes genetically evolved in-
stincts. Such objects are collected for the sheer plea-
sure of collecting them (not for any particularly good ex-
plicit and proximate reasons), and such pleasure is nearly
universal across human cultures. One of the immedi-
ate proximate motivations is decoration. According to
Dr. Mary C. Stiner, an archaeologist at the University of
15
Arizona, "Ornamentation is universal among all modern
human foragers."[W02] For an evolutionary psychologist,
such a behavior that has a good ultimate explanation, in
terms of natural selection, but has no proximate rationale
other than pleasure, is a prime candidate to be a geneti-
cally evolved pleasure that motivates the behavior. Such
is, if the reasoning in this essay is correct, the human
instinct to collect rare items, art, and especially jewelry.
Point (2) requires some further explanation. At first,
the production of a commodity simply because it is costly
seems quite wasteful. However, the unforgeably costly
commodity repeatedly adds value by enabling beneficial
wealth transfers. More of the cost is recouped every
time a transaction is made possible or made less expen-
sive. The cost, initially a complete waste, is amortized
over many transactions. The monetary value of precious
metals is based on this principle. It also applies to col-
lectibles, which are more prized the rarer they are and
the less forgeable this rarity is. It also applies where
provably skilled or unique human labor is added to the
product, as with art.
We have never discovered or made a product that does
really well on all three scores. Art and collectibles (in
the sense that word is used in modern culture, rather
in the technical sense it is used in this paper) optimize
(2), but not (1) or (3). Common beads satisfy (1) but
not (2) or (3). Jewelry, made at first out of the most
beautiful and less common shells but eventually in many
cultures out of precious metals, comes closer to satisfy-
ing all three properties. It is no coincidence that precious
metal jewelry usually came in thin forms such as chains
and rings, allowing for inexpensive assaying at randomly
chosen locations. Coins were a further improvement -
substituting small standard weights and trademarks for
assays greatly reduced the costs of small transactions us-
ing precious metals. Money proper was just a further
step in the evolution of collectibles.
The kind of mobile art also made by Paleolithic man,
(small figurines and the like) also matches these char-
acteristics well. Indeed, Paleolithic man made very few
objects that were not either utilitarian, or shared char-
acteristics (1)-(3).
There are many puzzling instances of useless or at least
unused flints with homo sapiens. We have mentioned the
unusable flints of the Clovis people. Culiffe[C94] dis-
cusses a European Mesolithic era find of hundreds of
flints, carefully crafted, but which micrograph analysis
reveals were never used for cutting.
Flints were quite likely the first collectibles, preceding
special-purpose collectibles like jewelry. Indeed, the first
flint collectibles would have been made for their cutting
utility. Their added value as a medium of wealth transfer
was a fortuitous side effect that enabled the institutions
described in this article to blossom. These institutions, in
turn, would have motivated the manufacture of special-
purpose collectibles, at first flints that need have no ac-
tual use as cutting tools, then the wide variety of other
kinds of collectibles that were developed by Homo sapiens
sapiens.
FIG. 10: Shell money from Sumer, c. 3,000 B.C.
During the Neolithic era, in many parts of the Mid-
dle East and Europe, some kinds of jewelry became more
standardized - to the point where standard sizes and as-
sayability were often valued over beauty. In commercial
areas the quantity of this jewelry sometimes greatly ex-
ceeded that of traditional jewelry in hoards. This is an
intermediate step between jewelry and coins, when some
collectibles increasingly took a fungible form. Around
700 B.C., the Lydian kings started issuing coins, as de-
scribed above. The unforgeable costliness of standard
weights of precious metals could now be "assayed" in
a marketplace, by wage earners, or by tax collectors via
trademark, i.e. trust in the mint’s brand, instead of chop-
ping coiled wire at a randomly selected spot.
It is no coincidence that the attributes of collectibles
are shared with precious metals, coins, and the reserve
commodities that have backed most non-fiat currencies.
Money proper implemented these properties a purer form
than the collectibles used during almost all of human
prehistory.
A novelty of the 20th century was the issue of fiat cur-
rencies by governments. ("Fiat" means not backed by
any reserve commodity, as the gold- and silver-based cur-
rencies of previous centuries were). While generally ex-
cellent as a media of exchange, fiat currencies have proven
to be very poor stores of value. Inflation has destroyed
16
FIG. 11: Silver ring and coil money from Sumer, c. 2,500 B.C.
Note the standard size of cross-sections. Many of the pieces
had a standard weight, ranging from one-twelfth of a shekel
to sixty shekels. To assay a ring or coil, it could be weighed
and cut at random locations. (Courtesy Oriental Institute,
University of Chicago)
many a "nest egg". It is no coincidence that markets in
rare objects and unique artwork - usually sharing the at-
tributes of collectibles described above - have enjoyed a
renaissance during the last century. One of our most ad-
vanced high-tech marketplaces, EBay, is centered around
these objects of primordial economic qualities. The col-
lectibles market is larger than ever, even if the fraction
of our wealth invested in them is smaller than when they
were crucial to evolutionary success. Collectibles both
satisfy our instinctive urges and remain useful in their
ancient role as a secure store of value.
XII. CONCLUSION
Many kinds of wealth transfers - one-way and mutual,
voluntary and coerced - face transaction costs. In vol-
untary trades both parties gain; a truly free gift is usu-
ally an act of kin altruism. These transactions create
value for one or both parties as much as the physical act
of making something. Tribute benefits the victor and a
judgment of damages can prevent further violence as well
as benefiting the victim. Inheritance made humans the
first animals to pass wealth to their next generation kin.
These heirlooms could in turn be used as collateral or
payment in trade for goods, for food to stave off star-
vation, or to pay a marriage bride price. Whether the
costs of making these transfers - transaction costs - are
low enough to make the transfers worthwhile is another
matter. Collectibles were crucial in making these kinds
of transactions possible for the first time.
Collectibles augmented our large brains and language
as solutions to the Prisoner’s Dilemma that keeps al-
most all animals from cooperating via delayed recipro-
cation with nonkin. Reputational beliefs can suffer from
two major kinds of errors - errors of about which person
did what, and errors in appraising the value or damages
caused by that act. Within clans (the small and immedi-
ately local kin group, or extended family, which formed a
subset of a tribe), our large brains could minimize these
errors, so that public reputation and coercive sanctions
superceded the limited motivation provided by the coun-
terparty’s ability to cooperate or defect in the future as
the main enforcer of delayed reciprocation. In both Homo
sapiens neanderthalis and Homo sapiens sapiens, with
the same large brain size, it is quite likely that every
local clan member kept track of everybody other local
clan member’s favors. The use of collectibles for trade
within the small local kin group may have been mini-
mal. Between clans within a tribe both favor tracking
and collectibles were used. Between tribes, collectibles
entirely replaced reputation as the enforcer of reciproca-
tion, although violence still played a major role in enforc-
ing rights as well as being a high transaction cost that
prevented most kinds of trade.
FIG. 12: When costliness becomes forgeable ? Glass trade
beads, manufactured in Venice in the 16th or 17th century,
excavated from Mali, Africa. Such beads were very popu-
lar wherever European colonialists encountered Neolithic or
hunter-gatherer cultures.
To be useful as a general-purpose store of wealth and
means of wealth transfer, a collectible had to be embed-
ded in at least one institution with a closed-loop cycle,
17
so that the cost of discovering and/or manufacturing the
object was amortized over multiple transactions. Fur-
thermore, a collectible was not just any kind of beauti-
ful decorative object. It had to have certain functional
properties, such as the security of being wearable on the
person, compactness for hiding or burial, and unforge-
able costliness. That costliness must have been verifiable
by the recipient of the transfer - using many of the same
skills that collectors use to appraise collectibles today.
The theories presented in this paper can be tested by
looking for these characteristics (or the lack of them)
in the "valuables" often exchanged in these cultures, by
examining the economic gains from the cycles through
which these valuables move, and by observing preferences
for objects with these characteristics in a wide variety of
cultures (including modern ones).
With its unprecedented technology of cooperation, hu-
mans had become the most fearsome predator ever seen
on the planet. They adapted to a shifting climate, while
dozens of their large herd prey were driven, by the hunt-
ing and the climate change in America, Europe, and Asia,
to extinction. Today, most large animals on the planet
are afraid of projectiles - an adaption to only one species
of predator [R97]. Cultures based more on gathering than
hunting also greatly benefitted. A population explosion
followed - Homo sapiens sapiens was able to populate
more parts of the planet and at a density over ten times
that of Homo sapiens neanderthalis [C94], despite weaker
bones and no increase in brain size. Much of this increase
may be attributed to the social institutions made pos-
sible by effective wealth transfer and language - trade,
marriage, inheritance, tribute, collateral, and the ability
to assess damages to dampen cycles of vengeance.
Primitive money was not modern money as we know
it. It took on some of the function modern money now
performs, but its form was that of heirlooms, jewelry, and
other collectibles. The use of these is so ancient that the
desires to explore, collect, make, display, appraise, care-
fully store, and trade collectibles are human universals
- to some extent instincts. This constellation of human
desires might be called the collecting instinct. Searching
for the raw materials, such as shells and teeth, and man-
ufacturing of collectibles took up a considerable portion
of many ancient humans’ time, just as many modern hu-
mans expend substantial resources on these activities as
hobbies. The results for our ancient forebears were the
first secure forms of embodied value very different from
concrete utility - and the forerunner of today’s money.
[1] [A90] Adams, Charles, For Good and Evil: The Impact
of Taxes on Civilization
[2] [A98] Tim Appenzeller, "Art: Evolution or Revolution?",
Science 282(Nov 20, 1998), p. 1452. See also the home
page of Stanley Ambrose
[3] [B04] The Blombos Cave Project
[4] [C94] Culiffe, Barry, ed., The Oxford Illustrated History
of Prehistoric Europe, Oxford University Press 1994.
[5] [D89] Dawkins, Richard, The Selfish Gene, Oxford Uni-
versity Press 1989.
[6] [D94] Davies, Glyn, A History of Money, From Ancient
Times to the Present Day, University of Wales Press
1994.
[7] [DW88] Daly, Martin and Wilson, Margo, Homicide, New
York: Aldine (1998).
[8] [G95] Gilead, I. 1995. "The Foragers of the Upper Pale-
olithic Period," in Archaeology and Society in the Holy
Land. Ed. by T. E. Levy. New York, Facts on File.
[9] [G01] [ref: http://www-
geology.ucdavis.edu/ GEL115/115CH1.html]
[10] [Gr01] Graeber, David, Towards an Anthropological The-
ory of Value, Palgrave 2001.
[11] [I98] Ifrah, Georges, The Universal History of Numbers,
John Wiley and Sons 1998, pg. 73.
[12] [K99] Kohn, M. and Mithen, S. "Handaxes: Products of
sexual selection?", Antiquity, 73, 518-526.
[13] [L94] Landa, Janet, Trust, Ethnicity, and Identity: Be-
yond the New Institutional Economics of Ethnic Trading
Networks, Contract Law, and Gift-Exchange, The Uni-
versity of Michigan Press, second edition, 1998.
[14] [M1892] Menger, Carl, "On the Origins of Money" Eco-
nomic Journal, volume 2,(1892) p. 239-55. translated by
C.A. Foley
[15] [M50] Mauss, Marcel, The Gift, 1950, English translation
by W.D. Halls, W.W. Norton 1990.
[16] [M93] (Morse 1993) via
http://www.wac.uct.ac.za/wac4/symposia/papers/s095wht1.pdf
[17] [R96] Riddley, Matt, The Origins of Virtue, Viking 1996.
[18] [T01] Taylor, Alan, American Colonies ? The Settling of
North America, Penguin 2001.
[19] [P89] Plattner, Stuart, Economic Anthropology, Stanford
University Press 1989.
[20] [W77] Wiessner, P. 1977. Hxaro: a regional system at
reciprocity for reducing risk among the !Kung San. Un-
published PhD thesis: University of Michigan.
[21] [W82] Wiessner, P. 1982. Risk, reciprocity and social in-
fluences on !Kung San economies. In: Leacock, H. R. and
Lee, R.B. (eds) Politics and history in band societies: 61-
84. London: Cambridge University Press.
[22] [W95] White, Randall, "Ivory Personal Ornaments of Au-
rignacian Age: Technological, Social and Symbolic Per-
spectives", Institute For Ice Age Studies,
[23] [W97] White, Randall, "From Materials To Meaning",
Institute For Ice Age Studies,
[24] [W98] Winterhalder, Bruce, "Intra-Group Resource
Transfers: Comparative Evidence, Models, and Implica-
18
tions for Human Evolution"
[25] [W02] Wilford, John, "Debate is Fueled on When Hu-
mans Became Human", New York Times, February 26th,
2002
> ***"money is a formal token of delayed reciprocal altruism" *** - R. Dawkins
Why is it called "Fiat"? What does the term "Fiat" mean?
https://en.wikipedia.org/wiki/John_Maynard_Smith
### Laffer curve:
The Laffer curve was first introduced introduce in 1974 by the American economist [Arthur Laffer](https://en.wikipedia.org/wiki/Arthur_Laffer).
The Laffer curve illustrates a theoretical relationship between revenue and taxation. It is used to illustrate that the more an activity is taxed, the less of it is generated. Inversely, the less an activity is taxed, the more of it is generated.
Learn more here: [Laffer Curve](https://en.wikipedia.org/wiki/Laffer_curve)
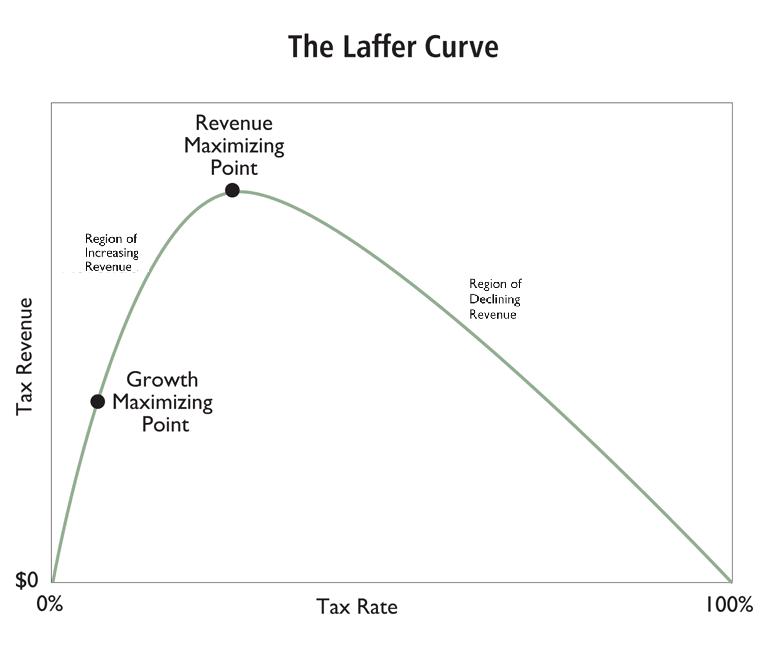
The creation of marriage as a contract between men and women came into being over time as early human communities settled on what was a "normal" way for them to organize and grow a family.
Marriage became the union between two people that was recognized by their community or their clans. The married couple stayed together and worked as a unit to feed and care for the children they produced.
Look up this source later.
how do i take a printout of this paper?
how do i print this paper?
Tribute may be the precursor to slavery since if you corral tribute payers the threat of violence for underproduction is ever-present - thus overcoming Laffer limitations - but balanced against maintenance costs.
Some examples of valued artifacts/collectibles:

*Figure 1: Wampum belt, with a white ground, and two parallel zig-zag lines of purple wampum, three beads in width.*

*Figure 2: Perforated Nassarius gibbosulus from archaeological layers dated to between 73,400 and 91,500 years ago at Taforalt.*

*Figure 3: Homo sapiens Artifacts from Blombos Cave, South Africa*
### Fun fact:
**Barter doesn't scale.** Imagine that you could barter on Amazon. Considering that Amazon currently sells around **500,000,000 different products** then it would need to keep track of:
$$2.5 \times 10^{17}$$
different prices.
### Velocity of money:
The velocity of money corresponds to the number of times one unit of money is spent to buy goods and services per unit of time. It corresponds to how much a unit of currency is used in a given period of time. Velocity is important in order to measure the rate at which money in circulation is used for purchasing goods and services.
Learn more here: [Velocity of money](https://en.wikipedia.org/wiki/Velocity_of_money)
Seashells from the Mediterranean and Atlantic coasts have been found in 30,000-year-old Sapiens sites in the European heartland. IT is likely that these shells got to the continental interior through long-distance trade between different human bands. Shell beads helped create and maintain exchange networks between coastal and inland tribes.
Learn more here: [Tiny Ancient Shells -- 80,000 Years Old -- Point To Earliest Fashion Trend](https://www.sciencedaily.com/releases/2009/08/090827101204.htm)

*Perforated Nassarius gibbosulus from archaeological layers dated to between 73,400 and 91,500 years ago at Taforalt.*
The author of this paper is Nick Szabo. He is polymath, computer scientist, legal scholar, and cryptographer known for his research in digital contracts and digital currency. N. Szabo developed the concept of "smart contracts" with the goal of bringing the "highly evolved" practices of contract law and practice for the design of electronic commerce protocols between strangers on the Internet. He designed Bit Gold, which many consider the precursor to Bitcoin.
You can learn more about Nick Szabo here:
- Twitter: [@NickSzabo4](https://twitter.com/NickSzabo4)
- Blog: [Unenumerated](http://unenumerated.blogspot.com/)
> ***"Money is accordingly a system of mutual trust, and not just any system of mutual trust: money is the most universal and most efficient system of mutual trust ever devised."*** - Y. N. Harari
#### Fiat currency:
Fiat money is currency that has no intrinsic value. It is declared by governments to be legal tender, but it is not backed by a physical commodity. The value of fiat money is derived from the relationship between supply and demand rather than the value of the material that the money is made of.
Learn more here: [Fiat money](https://en.wikipedia.org/wiki/Fiat_money)

*The Chao established in 1260 was the official banknote of Yuan dynasty is the earliest known form fiat money.*
### First human collectibles
Flints were likely the first human collectibles. They preceded special-purpose collectibles like shells, beads or jewelry. Flint collectibles had utility/functional value for their cutting utility. The added value as a medium of wealth transfer was a fortuitous side effect.
Learn more here: [Unenumerated - Artifacts of wealth: patterns in the evolution of collectibles and money](http://unenumerated.blogspot.com/2016/07/artifacts-of-wealth-patterns-in_15.html)

**The first coins in human history were created around 640 BC by King Alyattes of Lydia**, in western Anatolia. The Kingdom of Lydia existed from about 1200 BC to 546 BC and was one of the wealthiest empires of the ancient world and at its greatest extent, it covered all of western Anatolia.
Lydians were the first to introduce the concept of coinage. The Lydian coins were made of electrum an alloy with a standard weight of gold and silver, or pure silver and gold. The coins were imprinted with an identification mark, a design of lion and an ox.
The marks on the coins testified to two things:
1. it indicated how much precious metal the coin contained.
2. it identified the authority that issued the coin and that guaranteed its contents.
Almost all coins in use today are descendants of the Lydian coins.
Learn more here: [Lydia](https://www.ancient.eu/lydia/)

### Yap, the island of stone money
Another interesting form of money is the one found on the island of Yap, in the Pacific Ocean.
The Yapese use a form of stone money that they call Rai. Rai are large, very heavy, circular disks carved out of limestone that the Yapese brought back to Yap by the sea on their small bamboo boats.
They use Rai as a form of currency, or store of value and a piece of stone money is really valuable, you wouldn't use it for some everyday purchase.
Learn more here:
- [Rai stones](https://en.wikipedia.org/wiki/Rai_stones)
- [NPR - The Island Of Stone Money](https://www.npr.org/sections/money/2011/02/15/131934618/the-island-of-stone-money)

If you want to expand on this topic here are other interested resources:
- [Nick Szabo and Naval Ravikant discussing "What is money?" on the Tim Ferriss podcast (min 19:05)](https://www.stitcher.com/podcast/tim-ferriss-show/the-tim-ferriss-show/e/50383922)
- [This is American Life - The invention of Money](https://www.thisamericanlife.org/423/transcript)
- [NPR - The Island Of Stone Money](https://www.npr.org/sections/money/2011/02/15/131934618/the-island-of-stone-money)
For a given artifact to have value and be considered as a collectible it needs to have the following qualities:
1. It needs to be secure, hard to steel and difficult to lose.
2. It needs to be hard to copy.
3. Their value is easy to approximate with observations or measurements.
You can learn more here: [Artifacts of wealth: patterns in the evolution of collectibles and money](http://unenumerated.blogspot.com/2016/07/artifacts-of-wealth-patterns-in_15.html)

*Examples of artifacts as wealth*
Like the fur trade rendezvous
e.g. the Biblical story of Jacob (Israel) working for his brides.
Not discussed here is the importance of ornamentation for signaling fitness in mate selection. For example, wearing tiger teeth signals competency as a hunter while wearing hard-to-make jewelry signals excess time and competency as a gatherer. And the costlier the ornament, the better it signals fitness, while also serving as a bulwark against fraud. Humans selected one another for ornamentation and ostentatious spending.
### The Sungir children
In 1955 Archaeologists discovered a 34,000-year-old tomb in Sungir (Russia). This tomb belonged to a mammoth-hunting culture and contained two skeletons, a boy, and a girl. This tomb was particularly interesting because of the ornaments that it contained. The boy wore a fox-tooth hat and a belt with 250 fox teeth and was covered with 5,000 ivory beads. The girl was adorned with 5,250 ivory beads. Both children were surrounded by statuettes and various ivory objects.
***The 10,000 ivory beads, 250 fox teeth (at least 60 foxes had to be killed) that covered the two children, required at least some 7,500 hours of delicate work, well over three years of labor by an experienced artisan.***
Only cultural beliefs can explain why they received such an extravagant burial. One theory is that they owed their rank to their parents.
This shows that **30,000 years ago Sapiens could invent complex sociopolitical codes** that went far beyond the dictates of our DNA and the behavior patterns of other human and animal species.

### !Kung - Hxaro Exchange
The !Kung are a hunting-gathering society which occupy an area of the Kalahari Desert along the border between Namibia and Botswana. The !Kung are organized into small, nomadic bands. Due to their harsh environment, social relationships are important for survival. They maintain relationships and trade within their own small bands and with other nomadic bands through the Hxaro Exchange.
**The Hxaro Exchange is a delayed form of non-equivalent gift exchange.** When gifts are given from one member to another, the receiving party reciprocates the gesture by sending return gifts in the future, whether it be 6 months or 1 year. The gifts exchanged are usually not of equal value, which means that in order to balance out the value of the transaction the exchange needs to continue perpetually.
Learn more here:
- [Dobe ju'/hoansi](http://sc2218.wikifoundry.com/page/Dobe+ju%27%2Fhoansi)
- [Introduction to the Ju/'hoansi 's exchange system](http://sc2218.wikifoundry.com/page/INTRODUCTION+TO+THE+JU%2F+%27HOANSI+%27S+EXCHANGE+SYSTEM)
### TL;DR
- Humans evolved in small, largely self-sufficient, and mutually antagonistic tribes
- Reciprocal altruism is severely limited by unreliable memory. Who do I owe what? How much do I need to reciprocate?
- Collectibles helped reduce the need for favor-tracking.
- New ways of human cooperation were made possible thanks to the trade of collectibles.
- Human institutions like trade, marriage, inheritance or collateral were made possible thanks to effective wealth transfer.
- No other species is capable of such complex forms of reciprocal altruism.
- The evidence indicates this new development had matured by 40,000 B.P.