Particles having energies above $10^{18}$ eV are the so-called ultra-high-energy cosmic rays (UHECR). The UHECR are the most energetic particles known in the Universe. Spectrum of UHECR as observed by HiRes, TA and Auger experiments (as labeled). One can clearly observe a drop in the spectrum for Cosmic Ray energies above $10^{20}$ eV. K. Greisen's prediction was experimentally observed by three different experiments.  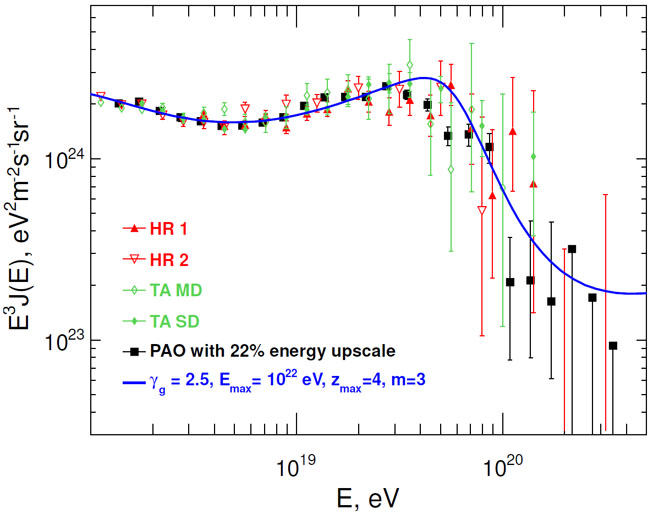Today the upper limit on the energy of Cosmic Rays is called **Greisen–Zatsepin–Kuzmin limit (GZK limit)**. Several months later G. Zatsepin and V. A. Kuzmin published [Upper Limit of the Spectrum of Cosmic Rays](http://www.jetpletters.ac.ru/ps/1624/article_24846.pdf). The Cosmic Microwave Background (CMB), discovered in 1966 by A. Penzias and R. Wilson, corresponds to the remnant photons of the Big Bang and acts like a black body spectrum with temperature T$_{\mathrm{CMB}} \simeq$ 2.73 K. At the highest energies, the Universe is no longer transparent to Cosmic Rays, that start interacting with the CMB photons.  The main reactions causing energy losses while the CR propagation are: * Compton interactions of nuclei * Pair production * Photodisintegration of the nucleus * Photopion production In the case of UHECR the reaction that has the most influence on the spectrum is photopion production given by the following interactions: \begin{eqnarray*} p +\gamma_{\text{CMB}} &\longrightarrow& \Delta^+ \longrightarrow p + \pi_0\\ p +\gamma_{\text{CMB}} &\longrightarrow& \Delta^+ \longrightarrow n + \pi^+\\ p +\gamma_{\text{CMB}} &\longrightarrow& \Delta^{++} +\pi^- \longrightarrow p + \pi^+ + \pi^-\,\, \end{eqnarray*}where $p$ is the UHECR and $\gamma_{\text{CMB}}$ are the CMB photons. Spectrum of cosmic rays as a function of their energy given by several different experiments.  We can easily determine the distance scale for energy loss given by:\begin{eqnarray*}\lambda_{E}=\frac{1}{\kappa \rho_{\gamma} \sigma_{\text{p}\gamma}}\end{eqnarray*}where $\kappa$ is the inelasticity (fraction of the initial energy available for the production of new particles), $\rho_\gamma$ is the CMB photon density, and $\sigma_{\text{p}\gamma}$ is the mean p-$\gamma$ interaction cross section. Using these values we find that $\lambda_{E}\simeq 10^{25}\,\textrm{cm}\simeq 3.24\, \textrm{Mpc}$. For each distance $\lambda_{E}$ the CR will loose 22% of its energy due to interactions with the CMB. The distance obtained corresponds to a characteristic time scale for energy loss of the order of $10^{15}$ s which is several orders of magnitude smaller than the age of the Universe ($4.3\times10^{17}$ s). This result could indicate that super GZK CRs: * There are near sources that we have not identified yet. Protons arriving at Earth with super-GZK energies must come from sources situated at less than $\sim 100\,Mpc$ away. * At the cutoff, the “visible” universe shrinks to a sphere of a few tens of Mpc of radius. This feature should be reflected in the energy spectrum of cosmic rays as a sharp drop. As a simple exercise, one can calculate the threshold energy of protons (we consider the UHECR to be a proton) for the photopion production. It is easy to obtain the mean energy for the CMB. We know that the CMB has a temperature of $T_{\gamma} \simeq \, 2.73$ and a mean wavelength of $\lambda_{\gamma}= 1.96\,mm$, from which we can compute the mean energy as $E_{\gamma} = hc/\lambda_{\gamma} \simeq 6.34 \times 10^{-4} \, eV$. Considering the proton and photon 4-momenta we have that the energy in the center of mass (CM) is given by: \begin{eqnarray*} \label{gzk1} \left( p_{\text{p}} + p_{\gamma} \right)^{\mu}\left( p_{\text{p}} + p_{\gamma} \right)_{\mu} &=& m_{\text{p}}^2c^2 + 2\frac{E_{\text{p}}E_{\gamma}}{c^2}-2\vec{p}_{\text{p}}\cdot\vec{p}_{\gamma} \end{eqnarray*}where $E_{\text{p}}$ is the energy, $m_{\text{p}}$ is the mass, and $\vec{p}_{\text{p}}$ is the three-dimensional momentum of the proton in the laboratory frame, and similarly for the photon. We can write the equation:\begin{eqnarray*} \label{gzk2} \left( p_{\text{p}} + p_{\gamma} \right)^{\mu}\left( p_{\text{p}} + p_{\gamma} \right)_{\mu} &=& m_{\text{p}}^2c^2 + 2\frac{E_{\text{p}}E_{\gamma}}{c^2} \left( 1- \cos{\theta} \right) \end{eqnarray*}where we have used the fact that protons are ultrarelativistic so $\beta \approx 1$. To find the threshold energy of this reaction we use the above equations and we assume a frontal collision $\theta=\pi$. We know that the threshold energy in the center of mass for this reaction is given by $\sqrt{s}=\left(m_{\text{p}}+m_{\pi^0}\right)c$. Finally we have that the threshold energy for the protons is given by:\begin{eqnarray*} \label{gzk3} 4\frac{E_{\text{p}}E_{\gamma}}{c^2} + m_{\text{p}}^2c^2 &=& \left( m_{\text{p}} + m_{\pi^0} \right)^2c^2 \end{eqnarray*}and the solution to of this equation for $E_{\text{p}}$ is:\begin{eqnarray*} \label{gzk4} E_{\text{p}} &=&\frac{m_{\pi^0}\left( 2m_{\text{p}} + m_{\pi^0} \right)c^4}{4E_{\gamma}}\,\,. \end{eqnarray*}Using the mean energy for the CMB we find that $E_{\text{p}}\simeq 1.07\times 10^{20}$ eV. We have found that protons with energies equal or higher than $10^{20}$ eV will interact with the CMB thus losing part of their energy.